

All scattering techniques have in common that a beam of radiation interacts with the structure of a sample. The beam can consist of electromagnetic radiation (photons, including x-rays) or quantum-mechanical particle waves such as neutrons or electrons. The incident beam is characterised by its energy, $E_0$, in J, and its wave vector, $\vec{k}_0$, in $\mathrm{m^{-1}}$.
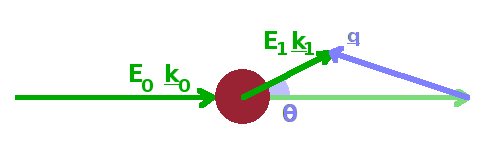
Following the scattering event, the energy of the scattered wave is $E_1$ and its wavevector $\vec{k}_1$. The momentum transfer, $\vec{q}=\vec{k}_1-\vec{k}_0$, in $\mathrm{m^{-1}}$, is the vector difference between the two wave vectors. The name comes from the fact that the momentum of a particle wave is proportional to its wave number, $p=\hbar k$. During any scattering event, momentum conservation $$\hbar\vec{q}=\hbar(\vec{k_1}-\vec{k_0})$$ as well as energy conservation $$\hbar\omega=E_1-E_0$$ have to be maintained, i.e. any energy or momentum gained or lost by the wave must have been exchanged with the structure of the scattering medium. During elastic scattering, $|\vec{k_1}|=|\vec{k_0}|$, no energy is transferred, and the wave number of the scattered wave and the incident wave are identical. Therefore, the momentum transfer reflects only the change in direction of the wave due to the scattering event (scattering angle). In inelastic scattering on the other hand, both energy and momentum are transferred, and the length as well as the direction of the scattered wave vector are different from the incident wave vector.
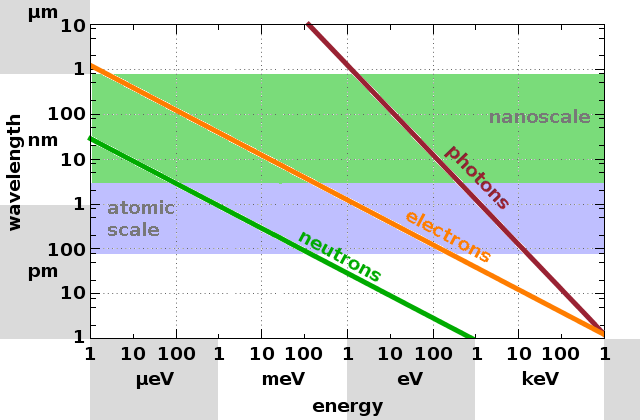
While both electromagnetic and particle waves can be used for scattering experiments, there is an important difference in the relationship between wavelength and energy for both types because particles have a rest mass. For x-rays (photons) the wavelength is simply the ratio of the speed of light and its frequency, i.e. $$\lambda_{\mathrm{photon}}=\frac{c}{\nu}=\frac{hc}{E}\qquad.$$ For neutrons on the other hand, the de Broglie wavelength is calculated from the momentum, which includes the rest mass of the particle: $$\lambda_{\mathrm{neutron}}=\frac{h}{m_nv}=\frac{h}{\sqrt{2Em_n}}\qquad.$$ As a result, the slope of the line representing the wavelength as a function of energy in a logarithmic plot is twice as steep for photons as it is for particle waves. The relationship for electrons is the same as for neutrons, but since their rest mass is only about one two-thousandth of the neutron rest mass, the same energy produces a wavelength about forty times as large.

Diffraction experiments probe structure of the order of the wavelength of the probe ray, while diffuse scattering probes structures significantly larger than the wavelength. Therefore, x-rays can be used for diffraction at atomic lattice planes or to probe nano-structure by diffuse scattering. Visible light can be diffracted at periodic structures on a sub-micron lengthscale, as is evident in opalescent materials. Since the wavelength of neutrons can be adjusted via their velocity, they, like x-rays, can diffract at atomic structures or scatter diffusely at nano-structures. Electron diffraction is rarely used as a stand-alone technique but is sometimes used in conjunction with transmission electron microscopy, using the same instrument but a different optical configuration.
The scattered intensity recorded by a detector in a scattering experiment is the product of five factors, $$\Delta I=\frac{\partial^2\sigma}{\partial\Omega\partial E}NI_0\Delta E\Delta\Omega\qquad:$$ The energy of the scattered wave, $E$, the solid angle, $\Omega$, captured by the detector, the incident intensity, $I_0$, coming from the source, the number of scattering objects, $N$, in the sample, and the scattering cross section, $\sigma$. The latter describes how likely a scattering process is, given all the ingredients listed above are present, i.e. the efficiency of the scattering process. A particular scattering experiment will be using monochromatic radiation and an angle-resolving detector; therefore we need to consider how the cross section changes with these parameters, i.e. use the differential scattering cross section, $\frac{\partial^2\sigma}{\partial\Omega\partial E}$.
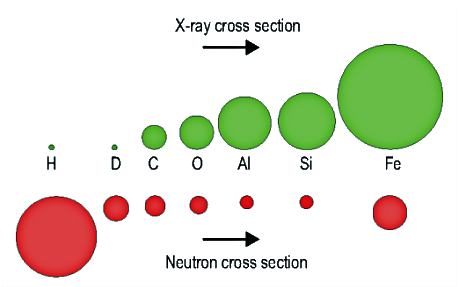
There are two contributions to this quantity: $$\frac{\partial^2\sigma}{\partial\Omega\partial E}=\left(r_0^2|f(q)|^2\right)S(\vec{q},\omega)\qquad,$$ one reflecting the efficiency of the individual scattering elements and the other their spatial arrangement. For x-rays, the atomic form factor, $f(q)$, represents the strength of the interaction between an incident wave and the electron cloud of an atom in the sample. It is multiplied by the classical electron radius, $r_0$. Because of the shape of the electron cloud (which is, in turn, determined by the shape of the occupied electronic states of the atom), the atomic form factor depends on the scattering angle and on the number of electrons in the atom. In the limit of zero angle (zero momentum transfer), the atomic form factor becomes simply the atom number in the periodic table, $$f(0)=Z\qquad.$$ Since neutrons interact with the nuclei rather than with the electron cloud of atoms, the scattering length, $b$, is used for neutrons instead of the atomic form factor term, $r_0|f(q)|$. There is no relationship between the position of an element in the periodic table and its neutron scattering length. In fact, protons are the most effective scatterers of all nuclides. Since the scattering length is a property of the nucleus rather than the atom, it is generally different for different isotopes of the same element. It is even possible for scattering lengths to be negative. Because of these differences, scattering cross sections for x-rays and neutrons can be very different for different samples, or even for different structures in the same sample.
The second component of the differential scattering cross section is the structure factor, $S(\vec{q},\omega)$. Determining this quantity is the goal of any scattering experiment, because its double Fourier transform in space and time is the correlation function, $G(\vec{r},t)$, giving the spatial distribution of whatever feature it is that causes the wave to scatter - atoms in the case of diffraction, nano-structures such as grains or layers in the case of diffuse scattering.
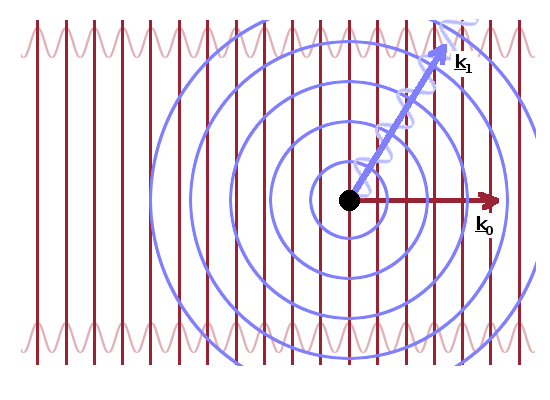
Because the incident beam is collimated by passing through a system of slits, the rays contained in it are all essentially parallel. The incident wave is therefore a plane wave, $${\rm e}^{{\rm i}\vec{k}_0\vec{r}}\qquad.$$ On the other hand, the scattered wave is a spherical wave, $$r_0f(q)\frac{{\rm e}^{{\rm i}\vec{k}_1\vec{r}}}{r}\qquad,$$ whose amplitude is given by the atomic form factor term, $r_0f(q)$, or the scattering length, $b$, for neutrons. A detector will see the superposition of these two waves.
As we have seen, the scattering of electromagnetic radiation by electron clouds and the scattering of neutrons by atomic nuclei can be treated in exactly the same way to reveal the structure of the sample (via the structure factor, $S(q,\omega)$) even though the interactions causing the scattering event are very different. We will next look at x-ray and neutron sources before moving on to experimental scattering techniques using either source to reveal different aspects of structure and morphology.