Condensed Matter Physics

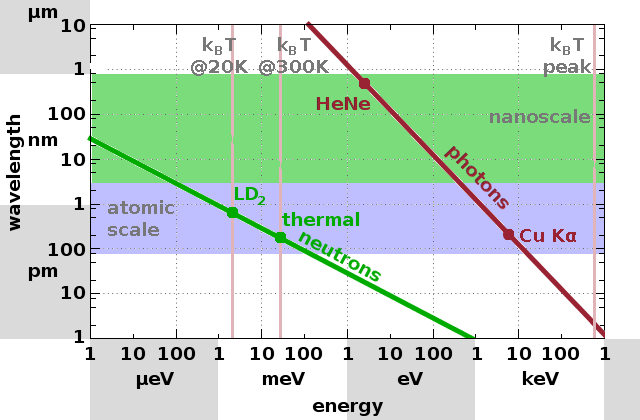
Apart from characteristic lengths on the atomic scale, diffuse scattering can also reveal nanostructures. The relevant techniques are small-angle neutron scattering (SANS), small-angle x-ray scattering (SAXS) and static light scattering (SLS), depending on whether neutrons, x-rays or visible light is used as the probing beam.

Waves (electromagnetic or particle) interact with structures of the same size as their wavelength (diffraction) as well as larger structures (diffuse scattering). The Fig. indicates what kind of structures are accessible (the area above the lines for photons or neutrons, respectively) for a given type of beam. Diffraction and diffuse scattering processes occur simultaneously and we can only distinguish them due to their different angle dependence. Since scattering takes place in reciprocal space, large structures cause scattering at small angles and vice versa given that there is a Fourier relationship between reciprocal and direct space.

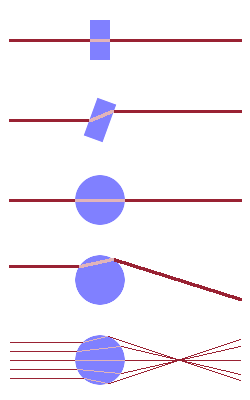
Unlike diffraction, which is a result of the constructive interference of waves scattered by periodic structures, diffuse scattering is simply a consequence of the refraction of rays at interfaces of different optical density. If a ray cuts through an interface at right angles, it will not be refracted. In the case of a flat object (e.g. a piece of sheet glass), there will be no refraction either when the ray enters or when it exits the object - the beam goes straight through (provided the object is transparent). If the ray hits the sheet at an angle, it will be refracted, but the deflection at the entrance and exit points just cancel out - a laser ray shone at a window will be offset sideways but the incident and refracted rays are parallel.

Substituting the flat object with a curved one such as a sphere changes this. If the ray hits the sphere at its equator, the incidence is normal and the beam will pass through without any refraction. However, if the ray enters the sphere away from the equator, it will be refracted, and the refracted ray will leave the sphere at a different angle because of its curvature. As a result it will be refracted back by more than it was refracted on entering, leading to a lensing effect. If the sphere is subjected to many parallel rays, this leads to a distribution of angles under which rays leave the sphere. The smaller the sphere, the larger is its curvature and therefore the wider the angular spread.

Therefore, we can measure the size of a scattering object by measuring the angular spread of the scattered rays emerging from the sample. It is important to note that it doesn't matter whether the scattering object has a smaller or larger optical density than its surroundings since small-angle scattering always measures the result of two coupled refraction processes (when the ray enters and when it leaves the scattering object). Therefore, it is impossible to distinguish between particles and pores in the sample.

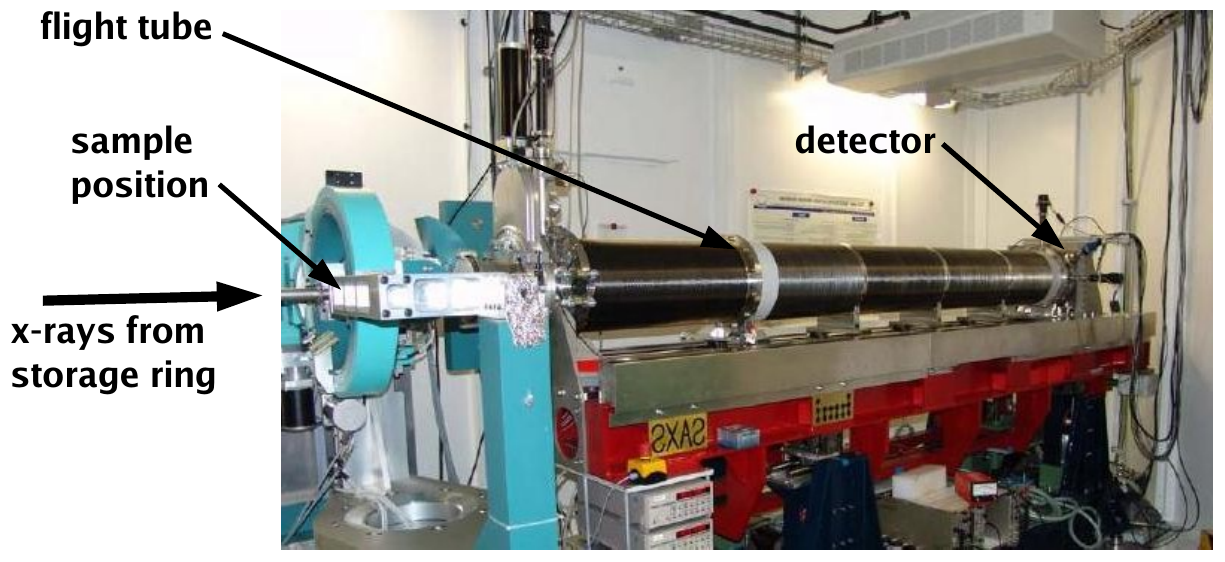
A SAXS instrument at a synchrotron x-ray source begins with a bending magnet or insertion device in the storage ring, where x-rays are generated by deflection of moving charges in a magnetic field. Since monochromatic radiation is needed in order to be able to relate the momentum transfer, $$q=\frac{4\pi}{\lambda}\sin{\theta}\qquad,$$ to the scattering angle, $\theta$, the beam passes through a monochromator, which selects one particular wavelength (energy) using a very sharp Bragg reflection. The beam is then passed through a collimator, i.e. a system of slits which limits the divergence of the beam. The beam can also be focused on either the sample or the detector using focusing elements such as curved mirrors or Fresnel zone plates. The thus conditioned beam is delivered to the sample position, after which there is a secondary beam tube of variable length with a two-dimensional detector at its end. Given that the size of the detector is fixed (for a given instrument), the sample-detector distance (or camera length) determines the angular range covered as well as the angular resolution.

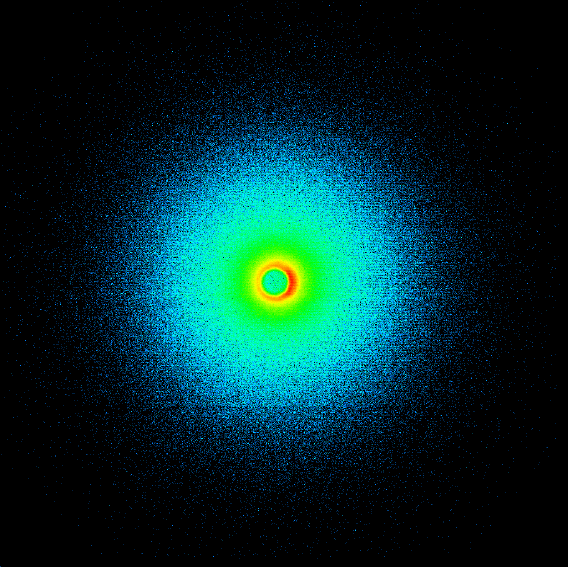
A typical SAXS pattern recorded using a two-dimensional detector is shown in the Fig. The count rate is highest at the centre, where those rays that are transmitted through the sample without refraction reach the detector. It decreases very rapidly radially outwards from the centre. The detector has to be protected from the intense primary beam using a beam stop, which shows as a round shape with reduced count rate (green circle in the Fig.) surrounding the centre of the pattern.

In most cases, SAXS patterns are radially symmetric - either because the scattering objects themselves are radially symmetric or because they are randomly distributed such that the different orientations are averaged. Therefore, the two-dimensional pattern can be radially averaged, producing a plot of count rate vs. momentum transfer (or scattering angle) such as the blue curve in the Fig. . The centre of the 2D pattern is on the left of the 1D plot and the edges of the detector image correspond to the right side of the 1D pattern. The count rate is colour-coded in the 2D image and shown numerically on the $y$-axis in the 1D plot. Note the logarithmic axes!

Such a reduced SAXS pattern contains essentially two pieces of information about the sample, and they are contained in two different sections of the curve, represented by the two fit lines shown in red. The Guinier regime (A) is the part of the pattern where a transition occurs from a near constant count rate onto a slope which decreases strongly as the scattering angle (momentum transfer) increases. The count rate in this regime can be described by the function $$I(q)\propto\exp{\left(\frac{R_g^2q^2}{3}\right)}\qquad,$$ where $R_g$ is the Guinier radius, which approximates the radius of gyration, i.e. the radius of a sphere in which a scattering object (whatever its shape is) may rotate freely. Further out lies the Porod regime (B), which describes the slope of the curve in terms of a power law (i.e. a straight line in the log-log plot): $$I(q)\propto q^{-n}\qquad.$$ The exponent $n$ characterises the surface morphology of the scattering object: a smooth interface will produce a slope of $n=4$, while less steep slopes indicate an interface that can be described as a fractal structure.

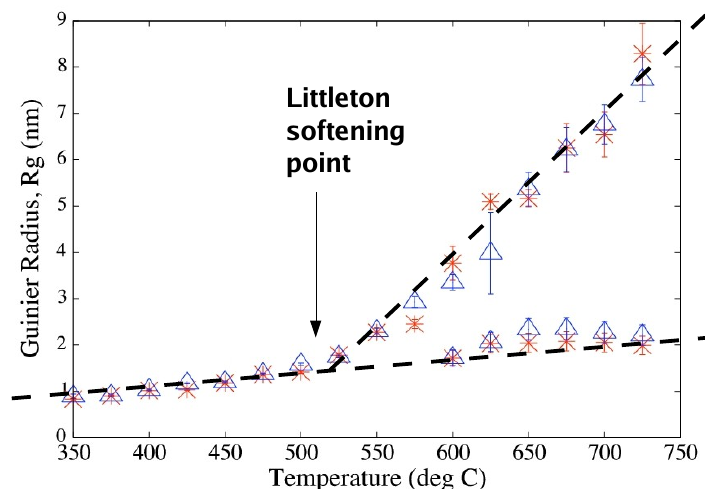
Individual exposures in x-ray scattering experiments are quite fast, so it is possible to do time-resolved experiments to investigate processes in situ. The case study is an example of this; it is an investigation in which a ceramic is formed by sintering alumina and zirconia nano-particles into a glass matrix. The three components are fine powders and are pressed into thin pellets, which are mounted in a furnace with windows transparent to x-rays. The furnace is placed on the sample table of the SAXS beamline. The temperature is gradually increased from $\mathrm{350^oC}$ to $\mathrm{725^oC}$ while SAXS patterns are recorded. As the raw data Fig. (top) shows, the Guinier regime shifts from right to left as the temperature rises. This indicates that the particle size is increasing gradually - the Fourier relationship between direct and reciprocal space means that a feature occurring at a smaller angle (momentum transfer) is a larger feature in direct space.

Above $\mathrm{500^oC}$, a second Guinier shoulder appears, which can be interpreted as a second characteristic length scale corresponding to a type of scattering object not observed at lower temperature. This co-incides with the softening point of the glass matrix used in the experiment - the softening point is the temperature at which a glass becomes just runny enough that it can be worked by a glass blower. When plotting the Guinier radii determined from the SAXS patterns, it appears that there is a gradual and very even increase of the primary particle size, with a much faster increase of the size corresponding to this second feature only present at the higher temperatures.

At the highest temperatures, the raw data curves show a maximum near to the small-angle end of the data set (on the left). The Guinier formula doesn't allow for this behaviour as it predicts an asymptotic limit for $q\to 0$. Such as shape is best modelled by a structure factor, i.e. by scattering objects whose positions in the sample aren't random but more regular. The structure factor maximum corresponds to a correlation length, i.e. a typical or average distance between the scattering objects. This is exactly equivalent to the peaks observed in total scattering patterns, except that, owing to the small angle (momentum transfer), much larger length scales than atomic distances are observed.

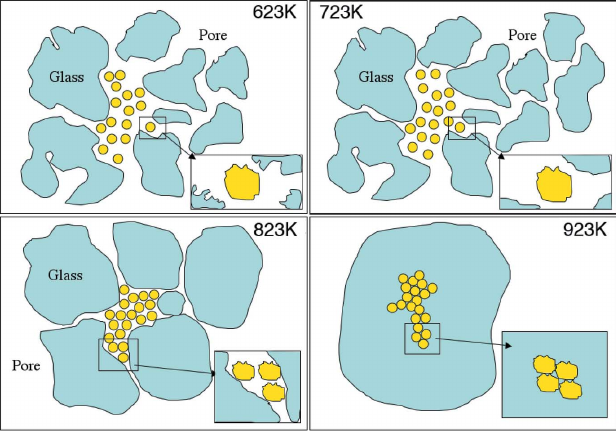
The aim of any scattering experiment is to derive a structural model of the material (and its evolution during an in-situ experiment). Taking all the evidence together, it is possible to conclude that the sample begins as a loose collection of individual nano-particles mixed up with larger, irregular glass particles. Around the softening point, the glass particles begin to change their shape and become more regular, with less rugged surfaces. As the glass blobs slowly flow and fill up the porosity, the nano-particles get pressed together and form aggregates, which give rise to the second, larger, Guinier feature. At the highest temperatures, the glass blobs join together, and the aggregates mark out the positions of the original triple junctions between adjacent glass particles, giving rise to a fairly regular spacing of these aggregates as reflected in the structure factor.