

So far, we have used the fact that ideal crystals show perfect translational order to describe their structure by identifying a repeat unit and a pattern to describe how exactly it is repeated to fill space. All this is based on perfect symmetry. In reality, crystals are neither ideal nor infinitely large. These deviations from the ideal structure as represented by the space group are called lattice defects.
Lattice defects can be classified according to their dimensionality as zero-, one- or two-dimensional defects. Point defects are zero-dimensional: an atom isn't where it is supposed to be according to the ideal description that we have, or it is the wrong type of atom. An isolated atom on the surface of a crystal can also be regarded as a point defect.
Dislocations are one-dimensional or linear defects: An additional lattice plane is inserted part-way into the crystal, causing a linear discontinuity at its end while the structure on either side of the line remains perfect.
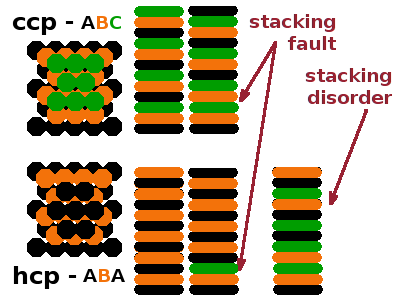
Grain boundaries separate small crystals which each can be described as perfect. A two-dimensional network of distortions is needed to adapt the misaligned lattices of the individual crystalline grains to each other. The surface of a finite crystal can also be seen as a two-dimensional defect, as can be stacking faults where the periodic repetition of layers, e.g. in a close-packed structure is disrupted.
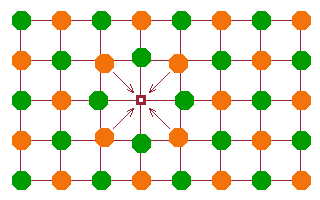
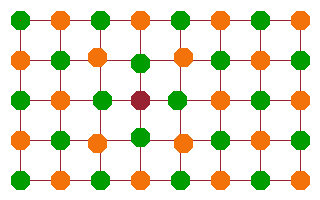
Perhaps the most obvious type of point defect is a vacancy: an atom is missing from its lattice point. This is illustrated in the figure by a purple square.
If the orange atoms are anions (negatively charged) and the green ones are positive cations, then a negative charge is missing in the lattice at the position of the vacancy. In other words, the vacancy has a positive charge relative to the lattice. As a result, nearby anions are drawn into the gap, and nearby cations are pushed away from it - they all adapt to the local distortion of the lattice potential.
The lattice as a whole doesn't collapse around the vacancy because the small adjustments to atom positions balance each other out.
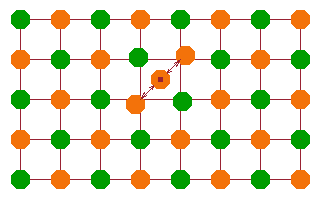
An interstitial atom is one that is located in between regular lattice positions. Of course, there is little space available, and the extra atom distorts the local potential quite dramatically. Nearby atoms are repulsed, particularly where like charges are involved.
Vacancies and interstitials are both highly mobile (see diffusion below). If one of each meet, they will usually annihilate, i.e. the interstitial atom will simply fill the vacancy and become a regular lattice atom. But, conversely, a vacancy-interstitial pair can be formed spontaneously if a phonon, a lattice vibration, supplies sufficient energy for an atom to be ejected from its lattice point.
This defect creation and annihilation mechanism means that at above-zero temperature, where there are always phonons active, a certain number of interstitials and vacancies will always be present: it is the crystal's way of absorbing thermal energy. The defect creation energy $E_{def}$ needed to form a pair depends on the particular material. If we know it and the thermal energy, $k_BT$, we can calculate the concentration of these intrinsic defects using the Boltzmann distribution: $$\frac{n}{N-n}\approx\frac{n}{N}=\exp{\left(-\frac{E_{def}}{k_BT}\right)}\qquad,$$ considering that the number $n$ of defects has to be small compared to the number $N$ of atoms in the crystal.
Impurities are the third type of point defect. A 'wrong' atom is placed on a regular lattice point. If the charge on the impurity atom is (even fractionally) different from that of a regular lattice atom, the lattice will be distorted. The picture shows a doubly charged cation (purple) on a site which is regularly occupied by a cation with a single charge (orange). The net result is a single extra charge relative to the lattice, which will attract the neighbouring (green) anions and repel the cationic neighbours.
| NaNa× | Na+ ion on a regular site |
| vO•• | oxygen vacancy, i.e. a missing O2- ion |
| Lii• | interstitial Li+ ion |
| CuNi' | Cu+ impurity on a Ni2+ site |
A common way of describing point defects is the Kröger-Vink notation. For each atom, its chemical symbol is given along with the chemical symbol of the atom that would normally occupy its lattice point and the charge relative to the lattice. Positive relative charges are indicated by dots, negative ones by dashes, and atoms that are neutral relative to the lattice are marked by a '×' symbol. Some examples are given in the table. The symbol for a vacancy or an interstitial is 'v' or 'i', respectively - always in lower case to avoid confusion with vanadium and iodine atoms or ions.
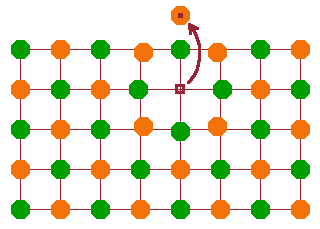
Compound defects are several point defects associated with each other. These can be transient or persistent, depending on whether their proximity stabilises or destabilises the crystal in terms of its free enthalpy.
A Schottky pair is a vacancy near the surface of a crystal, where the missing atom is placed on a regular lattice point on the surface. This is one mechanism by which vacancies are created. Since the atom, once it has found its surface site, is indistinguishable from other atoms on surface lattice points, there is no reason for the vacancy to remain at its position, so a Schottky pair is always a transient defect.
The Schottky pair formation mechanism can be described as $$\mathrm{A_A^{\times}+v_A'=v_A'+A_A^{\times}\qquad B_B^{\times}+v_B^{\bullet}=v_B^{\bullet}+B_B^{\times}}$$ where $\mathrm{A^+}$ is a cation, $\mathrm{B^-}$ an anion, and the second term on each side of the equation refers to an entity on the surface.
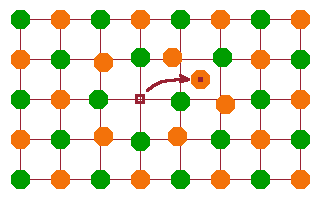
A Frenkel pair is an ion removed from its lattice position and located in an interstitial site, i.e. a combination of a vacancy and an interstitial: $$\mathrm{A_A^{\times}=A_i^{\bullet}+v_A'\qquad\qquad B_B^{\times}=B_i'+v_B^{\bullet}}$$ Frenkel pairs are created and annihilated continuously due to lattice vibrations - the higher the temperature, the more this happens. A Frenkel pair as such is transient; however, as both defects are highly mobile, it is likely that they will separate before they can annihilate.
The Schottky and Frenkel mechanisms constitute thermodynamic equilibria. The higher the temperature, the more the equilibrium will be biased towards the disordered side of the equation, favouring defects over regular lattice configurations. The Schottky and Frenkel equilibria ensure that there is always a minimum number of vacancies present in any crystal - the intrinsic vacancy concentration. Additional vacancies can be introduced by doping, i.e. by introducing impurities deliberately in order to create vacancies to balance the charge of the impurity relative to its location in the lattice.
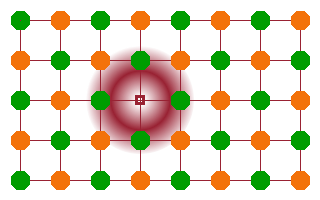

Another common type of compound defect is an electron trapped in an anion vacancy. In this case the electron balances the "missing" charge of the anion that would normally occupy the site in the regular lattice. This type of defect is known as an F-centre (from German Farbzentrum - colour centre) because of its tendency to produce vibrant colours in otherwise colourless or transparent materials. F-centres often occur when a material is exposed to ionising radiation: the radiation excites an electron from one of the atoms into the continuum, and the free electron is subsequently trapped by an intrinsic vacancy.
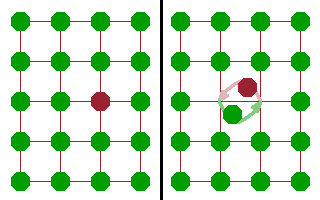
In ionic compounds ions are often highly mobile, which can give rise to a considerable ionic conductivity based on the diffusion of ions in a potential gradient. There are different mechanisms by which ions can move through a crystal lattice, but not all of them are equally efficient.
The most obvious mechanism is the rotary interchange mechanism, where two adjacent ions of the same species simply change places. In order for this to happen, both ions need to move simultaneously in a very confined space. Quite a lot of activation energy is required to enable the two atoms to move against the potentials defined by their neighbours.
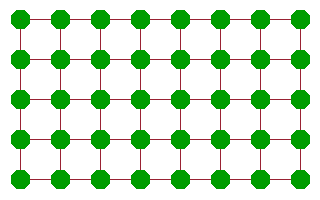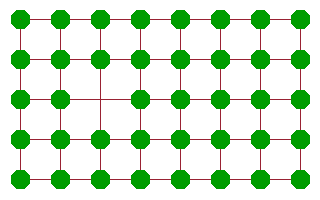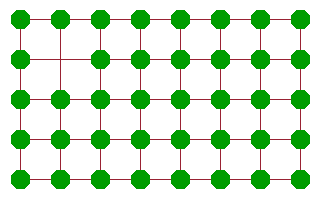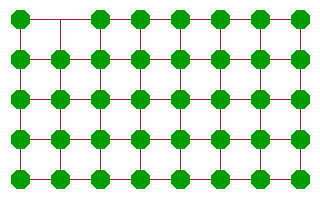
A more effective diffusion mechanism is vacancy diffusion, where an atom adjacent to a vacancy jumps into the vacancy, leaving its old site vacant in the process. While the atom will need to work against the potential of its neighbours to some extent, the amount of activation energy is much less than in the case of the rotary interchange because there is much more space for the atom to move in. Another atom further along can then jump into the new vacancy and continue the chain. The net result of these consecutive jumps is a motion of "the" vacancy across the crystal. Many ionic conductors are in fact conductors of vacancies of the corresponding site. An important example are oxygen vacancy conductors, which are often used in oxygen sensors or in fuel cells.

An alternative mechanism, which is also based on defects, is the interstitial diffusion mechanism. Here an interstitial is created (e.g. as part of an intrinsic Frenkel pair) and subsequently moves from one interstitial position to another. Since all the interstitial positions are equivalent, this motion is very effective because the activation energy needed to jump from one interstitial site (local potential minimum) to another can be recovered after each jump. This mechanism requires a little more energy than the vacancy mechanism but much less than the rotary interchange. It is particularly suited to small cations such as $\mathrm{Li^+}$ or $\mathrm{Na^+}$, whose mobility is often exploited in batteries and other electrochemical applications.
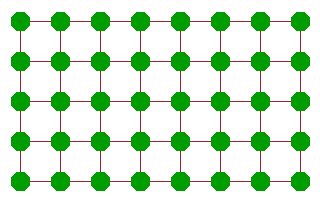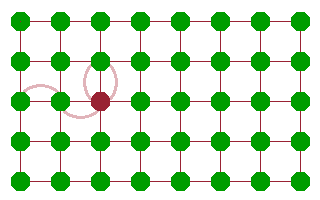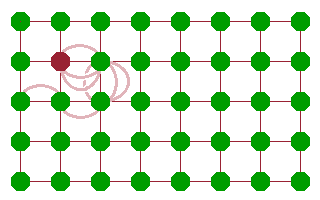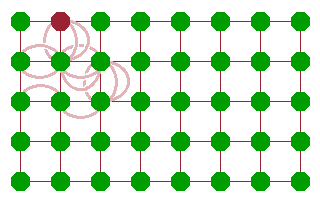
Whichever mechanism prevails, diffusion comes about as a sequence of jumps of atoms. The result is that some feature (an atom, ion or vacancy) moves through the crystal. This is often described in terms of a random walk, where individual jumps are uncorrelated - it is as likely for a mobile species to move back where it came from than to move to any other available neighbouring site. As a result, the distance travelled from a starting point scales with the square root of the number of jumps (and, assuming a constant jump rate, therefore, time).
The random walk model doesn't always describe the ionic conductivity found in fast ionic conductors such as those used in solid-state batteries properly. The reason for this is that the local potential surrounding a mobile species changes after a jump has occurred as the local configuration relaxes structurally towards thermodynamic equilibrium. More sophisticated diffusion models take this into account, e.g. by including a time-dependent probability for a reverse jump which diminishes over time as the original site relaxes. Such structural relaxation effects increase the probability for long-range motion and therefore increase the DC ionic conductivity of the material.

If an ionically conducting crystal is placed in a concentration gradient, there will be a net current of ions from higher to lower concentration. Since the ions carry charge, this corresponds to an electrical current proportional to the flux of particles, $j$. Fick's law quantifies this behaviour: $$j=\frac{{\rm d}N}{A{\rm d}t}=-D\frac{{\rm d}c}{{\rm d}z}\qquad,$$ where $N$ is the number of particles crossing a cross-sectional area $A$ per unit of time, $t$. This is linked to the concentration gradient $\frac{{\rm d}c}{{\rm d}z}$ perpendicular to $A$ by the diffusion coefficient, $D$, measured in m2/s. The diffusion coefficient (or diffusivity) is thermally activated: $$D=D_0\exp{\left(-\frac{E_a}{k_BT}\right)}\qquad,$$ with an activation energy, $E_a$, which is compared to the thermal energy available. For this reason, ionic conductivity increases with temperature (in contrast to electric conductivity in metals).
| conductor | anode | electrolyte | cathode | wire |
|---|---|---|---|---|
| electronic | $\checkmark$ | $\times$ | $\checkmark$ | $\checkmark$ |
| ionic | $\checkmark$ | $\checkmark$ | $\checkmark$ | $\times$ |
An application of directed diffusion in fast ionic conductors is the
lithium-ion battery.
A battery consists of a cathode, anode and electrolyte, which all need to have appreciable
ionic conductivity. The electrodes typically are
intercalation compounds,
i.e. materials with a layer structure where it is easy for the mobile ions to slide
between the layers, allowing many fast charge-discharge cycles without compromising structural
integrity. Nickel or cobalt oxide are frequently used in the cathode, while graphite
is a common anode material. The half-cell reactions taking place at the electrodes are thus:
cathode: LiNiO2 = NiO2 + Li+ + e-
anode: Li+ + e- + 6C = LiC6
Most modern lithium ion batteries use a lithium-conducting polymer as the electrolyte as these
materials tend to have the highest ionic conductivities while also being very lightweight.
When designing a battery, it is important to choose materials which have the required electrical
properties: the electrodes need to be both ionically and electrically conductive as it is here that
the conduction mechanism switches from electrons to ions and back. The electrolyte, on the other
hand, must be an ionic conductor with negligible electronic conductivity as an electronic current
between anode and cathode would short-circuit the battery.
Having established how point defects determine a material's conductivity, we'll next turn to dislocations and material strength, another aspect of defect structure having a major impact on material properties.