

The word group in the terms point group, plane group and space group has a specific meaning in mathematics. A group is a set of elements which share some characteristics, e.g. a finite set of numbers such as $\{1,2,3\}$, the infinite set of all integers, $\mathbb{N}$, or the contents of a fruit bowl, {apples, pears, oranges}. In order to form a group, the set must have an associated operation, e.g. addition, multiplication, or applying a symmetry operation.
Any group must have a number of properties with respect to how the operation is applied to the members of the group. First, the order in which operations are performed is immaterial as long as the order of the group members linked by the operations doesn't change. This is known as the associative property $$(A\ast B)\ast C=A\ast(B\ast C)\qquad.$$ For example, in the multiplication group of real numbers, $(2.0\cdot 3.0)\cdot 4.0=2.0\cdot(3.0\cdot 4.0)$. There must be exactly one neutral element among the group members such that $$A\ast E=E\ast A=A\qquad,$$ i.e. operating any member with the neutral element reproduces the same member. For example, for the multiplication group of real numbers, the neutral element is 1.0: $1.0\cdot n=n$ for any $n$. There must also be an inverse element for each element, i.e. $$A\ast A^{-1}=E\qquad.$$ In the case of the multiplication group of real numbers, $n$ and $\frac{1}{n}$ are inverse elements to one another: $3.0\cdot\frac{1.0}{3.0}=1.0$. This explains why there cannot be a multiplication group of integers - an inverse element could not be found within the group for each member. Finally, a group must have closure, i.e. the result of any operation on members of the group must be a member of the group itself. Unless the group is infinite in size, this will involve some cyclic property, i.e. applying the operation consecutively for a number of times will ultimately return the original operand.
A group is known as an Abelian group if it also has the commutative property, i.e. if operands can always be swapped without changing the result: $$A\ast B=B\ast A\qquad.$$ This is the case for multiplication groups ($2\cdot 3=3\cdot 2$) but not, for example, for groups whose operation entails a differential operator, $\frac{\partial}{\partial x}$.
For any group, a table can be written which contains the group members both in the first row and first column, and the result of the operation on these two members at each intersection. This is known as a multiplication table, even in groups where the operation isn't actually multiplication. For an Abelian group, commutativity results in the multiplication table being symmetrical about the diagonal. As each element has an inverse element and the group is closed, there are $n!$ bijective mappings for a group of $n$ members (i.e. a group "of order $n$").
A group needn't consist of numbers but can have other things as members. This is convenient when describing the relationship of the symmetry elements in a crystal structure: The list of symmetry elements (pure rotations, inversions, mirrors as well as screw axes, roto-inversions and glides) found in a crystal structure are the members of its point group. The operation associated with the group is the application of the respective symmetry element to the structure, turning the symmetry elements into symmetry operations.
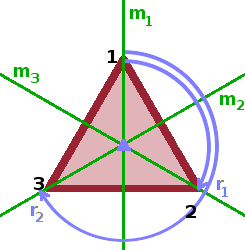
We will look at a simple symmetry group as an example: the dihedral group $S_3$. It describes a two-dimensional symmetry consisting of a three-fold rotation point and three mirror lines intersecting on the rotation point. The six symmetry elements making up the group can be represented by matrices listing the locations of points 1, 2 and 3 and how they are mapped after applying the symmetry element: $$e=\left(\array{1&2&3\\1&2&3}\right) ,\qquad m_1=\left(\array{1&2&3\\1&3&2}\right)$$ $$r_1=\left(\array{1&2&3\\2&3&1}\right),\qquad m_2=\left(\array{1&2&3\\2&1&3}\right)$$ $$r_2=\left(\array{1&2&3\\3&1&2}\right),\qquad m_3=\left(\array{1&2&3\\3&2&1}\right)$$
The elements include the three mirrors and a rotation by a third of a turn, two thirds of a turn or a full turn (the latter being the neutral element, $e$). The multiplication table of the group is a 6x6 matrix detailing how applying two symmetry operations consecutively reproduces the effect of one of the other symmetry elements. This is a general feature of all symmetry groups resulting from the closure property of groups.
Whenever two rotations are combined, the result is another rotation, two mirrors taken together lead effectively to a rotation, and combining a rotation with a mirror has the same effect as one of the mirrors. This relationship can easily be spotted in the multiplication table, which shows four sub-groups in its four quadrants.
This ability to predict the effect of applying different symmetries consecutively by reducing it to one of the individual symmetry elements present is what makes group theory useful to crystallographers. It makes it possible to identify easily all the locations of identical copies (mappings) of an atom based on the point group of the unit cell.
Having established a range of crystal structures and ways to describe them based on their symmetry, we will next turn to scattering theory to see how we can use beam interactions to probe structures and morphologies.