


Phase transitions occur in many branches of physics. They have in common that a more ordered and a more disordered form of the same material are in thermal equilibrium with one another. For a given pressure (such as ambient pressure), this can only be the case at one specific temperature, the phase transition temperature.
Phase transitions are driven by the difference in the free enthalpy, $G=H-TS$, of the two phases. At the transition, both phases have the same free enthalpy: $$\Delta_{\mathrm{tr}}G=\Delta_{\mathrm{tr}}H-T_{\mathrm{tr}}\Delta_{\mathrm{tr}}S\stackrel{!}{=}0\qquad,$$ i.e. the transition occurs when the entropy change at the transition outweighs the enthalpy difference due to the phase change. Since only the entropy term is explicitly temperature dependent, this defines a sharp transition temperature.
Given that entropy is a measure of disorder, it is clear that the more disordered phase is always the high-temperature phase at any phase transition.
Phase transitions occur whenever the kinetic energy, $k_BT$, of a phase overcomes the order sustained by its potential energy, where the latter can take different forms depending on the type of phase transition.

The examples that spring to mind first when considering phase transitions are the changes between the states of matter, i.e. melting and boiling.
At the melting point, a rigid crystalline structure transforms into a liquid, where interactions between atoms or molecules still exist but are flexible and insufficient to support a periodic structure.
At the boiling point, such permananent interactions between molecules or atoms outside of molecules disappear and individual particles move about largely independent of each other.
Therefore, the liquid phase is the ordered phase in relation to the boiling transition but the disordered phase in relation to the melting transition.

Phase transformations needn't involve the positions of atoms and the chemical bonds between them. For example, in the magnetic phase transition between a paramagnet and a ferromagnet, the atoms aren't displaced at all, but the orientation of magnetic moments, $p_m$, associated with them changes.
In the ferromagnetic state, the magnetic interaction energy, $p_mB$, is larger than the thermal energy $k_BT$. As a result, the individual moments align in a common fashion. As the temperature rises above the Curie temperature, $T_C$, the material changes into its paramagnetic state, where the individual moments are randomly orientated.
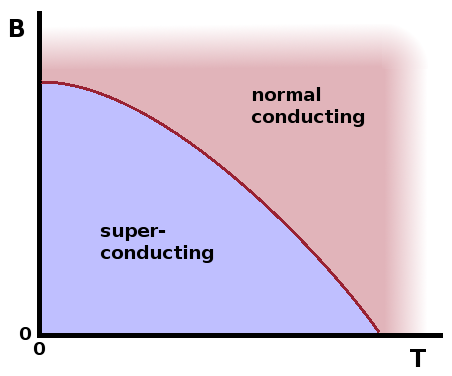
A superconductor has an ordered electronic state in the sense that electrons form Cooper pairs. Since the total spin of a Cooper pair has an integer value, the pairs are bosons and can therefore simultaneously occupy the lowest-energy state.
This ordering can be disrupted thermally in the same way the magnetic ordering in a ferromagnet can be overcome by thermal energy. At the same time, there is a critical magnetic field at which the superconductor turns into a normal conductor. As with any phase transition, this is a result of the free enthalpy of the superconducting state exceeding that of the normal-conducting state under these conditions. Critical field and temperature mutually depend on each other, just as boiling pressure and temperature do.
Crystallographic (aka structural) phase transitions are transitions between two different solid phases of the same material. As usual, the phase transition is driven by the free enthalpy of the two phases under the given conditions.

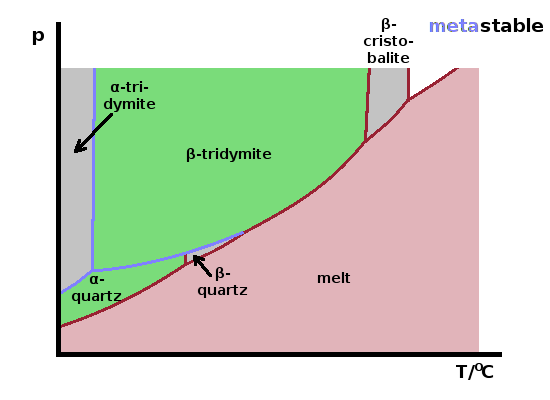
As an example, the phase diagram of silica, $\mathrm{SiO_2}$, is shown in the schematic. Cooling down slowly from the melt at ambient pressure results in a solid forming at the melting point, 1713oC. This solid is the mineral β-cristobalite. On reducing the temperature, again very slowly to allow the material to stay in thermodynamic equilibrium, the solid changes its structure, forming β-tridymite at 1470oC. There are two further structural phase transitions at 870oC and 573oC, where β-quartz and finally α-quartz form, respectively. All of these minerals are polymorphs of silica, i.e. they have the same composition but different structure. Only one of them can be thermodynamically stable under any given set of state variables (temperature, pressure etc.). There are a few other polymorphs of silica which only exist at much higher pressures.
The Greek-letter prefix distinguishes structurally very similar forms, usually in their order of discovery, although this often places the low-temperature, high-pressure forms at the top of the alphabet since these are usually more common minerals or easier to synthesise. To avoid this ambiguity, α-quartz and β-quartz are also known as low quartz and high quartz, respectively - low and high refer to temperature here.



The α-forms of tridymite and cristobalite aren't thermodynamically stable at any temperature at ambient pressure. However, they can exist indefinitely once formed under non-equilibrium conditions as metastable phases. These are essentially "frozen" non-equilibrium phases and can form if the material cools at a rate that is too fast for atomic re-arrangements to occur.
In contrast to the stable phases shown in a thermodynamic phase diagram, the conditions under which metastable phases form depend not just on state variables such as temperature and pressure but also the cooling rate, or, more generally, the rate at which energy is being removed from the system. A schematic metastable phase diagram of silica for a specific cooling rate is shown in the Figure.
Since geological processes occur across a wide range of pressures, temperatures and cooling rates, it is quite common for different polymorphs to exist as naturally occurring minerals. For example, cooling rates are very high during volcanic eruptions. On the other hand, metamorphic processes deep in the crust happen slowly but at high pressure.
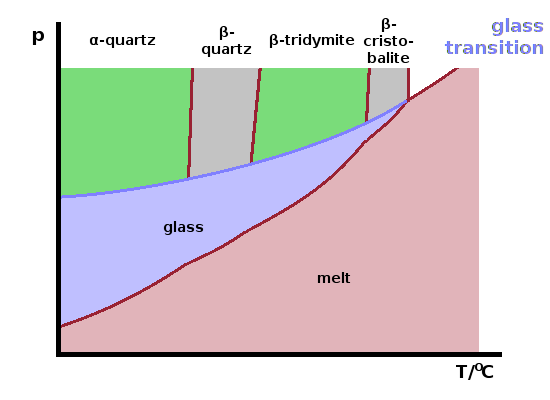
An extreme case of the formation of a metastable phase is the glass transition, where the melt is cooled ("quenched") at such a fast rate that the atoms haven't got enough time to arrange themselves into a periodic pattern. As a result, the disordered liquid structure is effectively frozen into an amorphous solid.
The local structure of an amorphous material is usually identical to that of a stable crystal of the same composition, i.e. the bond lengths, bond angles and co-ordination polyhedra are the same but the way they are linked together is different, with more disorder in the dihedral angles.
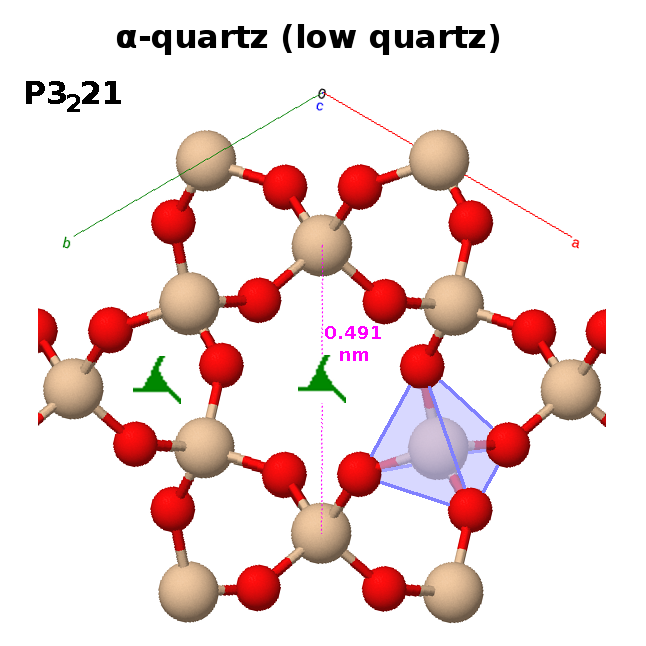
All of the polymorphs of silica (except for one of the high-pressure forms, stishovite) are made up of $\mathrm{SiO_4}$ tetrahedra connected via bridging oxygens.
In quartz, these corner-sharing tetrahedra form helices with a repeat unit of three tetrahedra. Therefore, a three-fold screw axis runs along the centre of each helix. Six such helices are arranged in a hexagonal pattern, which inherits the three-fold screw symmetry, leaving a gap in the middle.

The screw axis can define a clockwise ($3_2$) or anti-clockwise ($3_1$) helix, resulting in two structures which are mirror images of each other. They are enantiomorphs and energetically exactly eqivalent. As a result, quartz (and other such chiral structures) tend to form crystal twins, where two crystals with opposite chirality grow in different directions from the same nucleus.
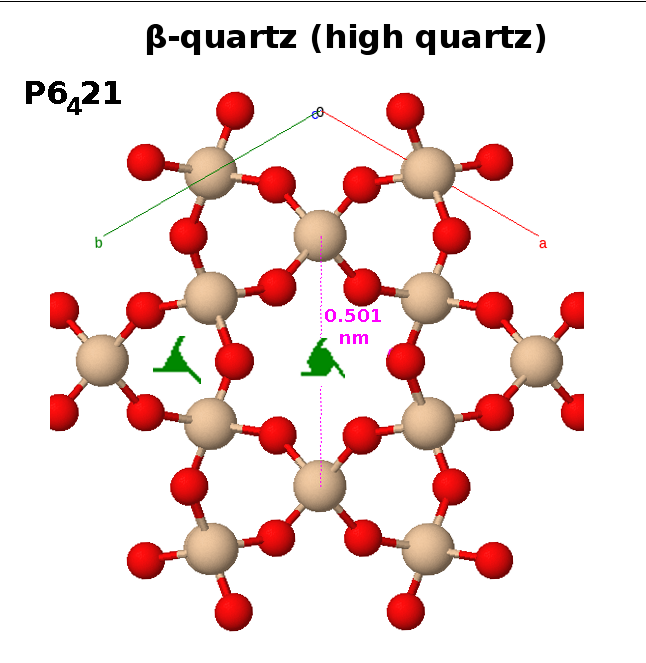
The structure of β-quartz is almost the same: The tetrahedra are still arranged in helices around a three-fold screw axis, and the helices remain grouped in a hexagonal pattern. The difference is that the structure is stretched a little along the $c$ axis until all the bridging oxygens end up at the same level at $\mathrm{z=\frac{1}{6}}$, $\mathrm{z=\frac{3}{6}=\frac{1}{2}}$ and $\mathrm{z=\frac{5}{6}}$. As a result, the symmetry of the hexagonal pattern increases to a six-fold screw symmetry with translations by one third of the lattice parameter on every turn ($6_4$ or $6_2$ depending on chirality).
The table below summarises structural features of the four polymorphs of silica which are stable at ambient pressure at various temperatures:
| $\mathrm{SiO_2}$ | α-quartz | β-quartz | β-tridymite | β-cristobalite |
| stable at | <573oC | 573-870oC | 870-1470oC | 1470-1713oC |
| Si-O-Si angle | 144o | 153o | 180o avg. (147o) | 180o avg. (147o) |
| density / $\mathrm{g\,cm^{-3}}$ | 2.65 | 2.53 | 2.25 | 2.20 |
| class | trigonal | hexagonal | hexagonal | cubic |

In tridymite and cristobalite, the motif of corner-sharing tetrahedra remains, but their helical arrangement is absent. The two structures are quite similar, deriving from the hexagonal and cubic forms of diamond, respectively, by inserting an oxygen atom between each of the silicon atoms on the carbon sites in the diamond structure. The forms which have a stability range at ambient pressure are the high-temperature (β) forms, where the inserted oxygen atoms appear to be centred between each pair of silicon atoms (Si-O-Si angle of 180o). This is due to motional averaging of two sites either side of the connecting line between the silicon atoms due to a rapid correlated twisting motion of adjacent tetrahedra. If we could take a very fast snapshot of a β-tridymite or β-cristobalite crystal, it would show a more conventional Si-O-Si angle of around 150o typical of oxygen because of its two lone electron pairs. In the α-forms, this motion is frozen and the usual Si-O-Si angle is actually observed, with a corresponding breaking of symmetry.
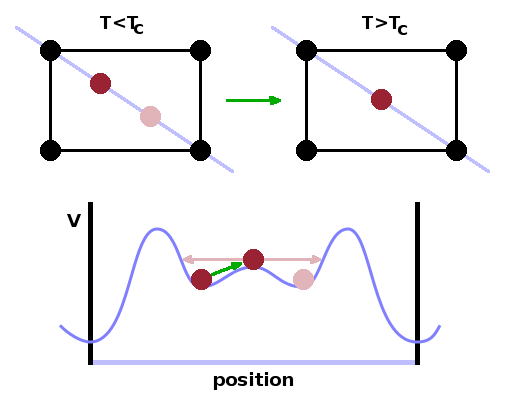
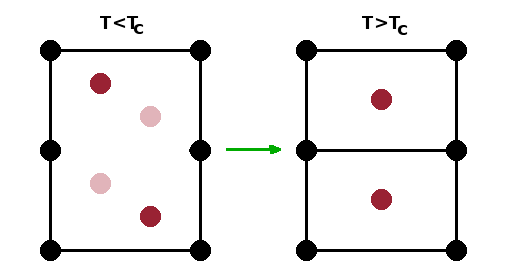
Phase transitions between polymorphs, particularly at low temperatures, frequently don't involve the breaking of bonds and therefore don't require large amounts of energy. This is the case when a small change in the position of some atoms in the unit cell is sufficient to change the symmetry of the structure, as in the case of the transition between the α and β forms of the different silica polymorphs discussed above. Such transitions are known as displacive phase transitions.
The simplified two-dimensional structure shown illustrates the principle of a displacive transition: Consider a crystal potential with two shallow minima inside the unit cell while the occupancy of these sites is ½. Below the phase transition, an ion doesn't have sufficient energy to overcome the small barrier between the two sites; the ion therefore remains trapped in either of the two sites. Above the transition, there is sufficient kinetic energy to overcome the internal barrier, giving the ion a wider potential trough to oscillate in, which is centred in the middle of the cell. Because of this motional averaging, the symmetry of the cell increases when heating the material above the phase transition temperature.
Displacive phase transitions can lead to the doubling of the unit cell due to symmetry breaking at the phase transition temperature. This occurs if atoms snap into different sites in adjacent unit cells upon cooling. Since unit cells, by definition, have to be identical, the low-temperature phase has an enlarged unit cell comprising two adjacent cells of the high-temperature phase.
| $\mathbf{T\lt T_c}$ | $\mathbf{T\gt T_c}$ | |
| temperature | low | high |
| pressure | high | low |
| symmetry | low | high |
| density | high | low |
| enthalpy | low | high |
| co-ordination | high | low |
Since the phase transition occurs when the free enthalpy $$G=U-TS+pV$$ of both phases is equal, we can see that temperature and pressure act in opposite directions. The symmetry is always lower in the low-temperature phase, and as a result its density is higher. The table summarises these relationships between the phases involved in a displacive phase transition.
Having established that phase transitions are a universal concept across many areas of physics and materials science, we will consider the thermodynamic variables characterising phase transitions in more detail.