

The number of lattices that can fill two- or three-dimensional space with periodically repeating units without leaving gaps or causing overlaps is limited. Therefore, there is a finite number of different crystal structures, and different crystalline solids may crystallise according to the same pattern. The metrics of the lattice may be different, but the symmetry is the same in such cases. Lattices which fill space without gaps are called Bravais lattices. There are five of them in two dimensions and 14 in three dimensions.
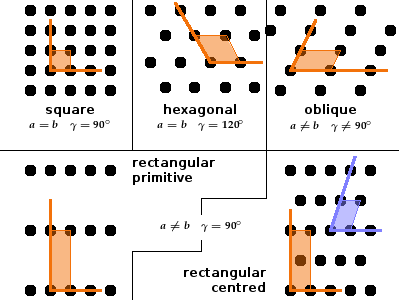
The most general and least symmetric Bravais lattice in two dimensions is the oblique lattice. If the angle between the two lattice vectors is 90°, the higher symmetry of the cell gives rise to a distinct Bravais lattice, either rectangular or square depending on whether the unit cell vectors have different length or not. In the case of a rectangular lattice, we can distinguish between a primitive rectangular lattice and a centred rectangular lattice, which has an extra lattice point (atom) at the centre. The centred rectangular lattice could be set up as a primitive lattice with lower symmetry (unit cell shown in green), but convention prefers the more symmetric description. Finally, if the lattice vectors are the same length and the angle is 120°, we have another special case with higher symmetry, the hexagonal lattice.
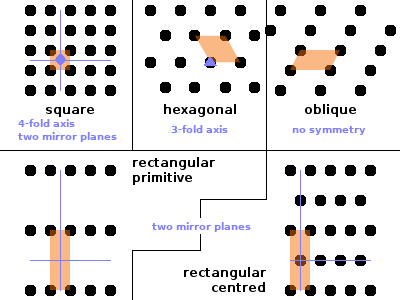
Clearly, the difference between the Bravais lattices boils down to symmetry. If the lattice vectors are at right angles, the unit cell can be folded over along lines intersecting either lattice vector at its centre: there are mirror lines crossing each pair of edges of the unit cell in the middle, and the two mirror lines intersect at the centre of the cell. This explains the special position of the central point in the centred rectangular lattice - it is located at the centre of symmetry of the cell and always maps onto itself under any symmetry operation.
In two dimensions, the effect of a mirror line and a rotation by 180° is the same, so the rectangular lattices both have a two-fold rotation axis at their centre. In the case of the square lattice, it is even a four-fold axis - turning the cell by 90° maps it onto itself because both lattice vectors are the same length. This is indicated by a green diamond shape in the diagram. There is also a four-fold axis in each corner of the cell: a 90° rotation around any of the lattice points again maps the lattice (though not the specific unit cell) onto itself. The hexagonal lattice has a three-fold symmetry by rotating in 120° steps around a lattice point, indicated by a triangular marker.
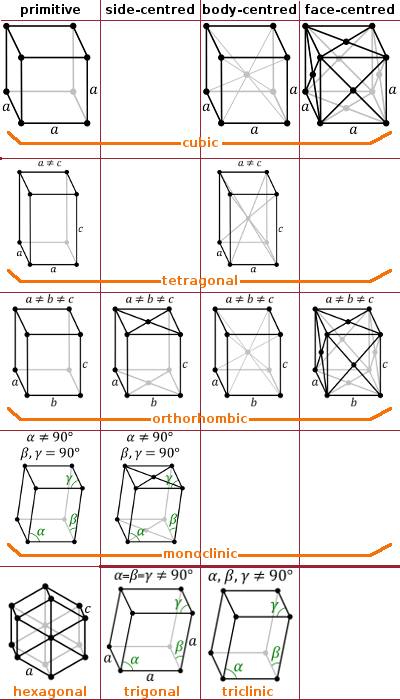
The same symmetry principles apply in three dimensions. The concept of a centred lattice expands into three distinct cases, depending on whether the additional point is at the centre of the unit cell (body-centred), of one face and, because of the translational periodicity, its opposite (side-centred) or on all its faces (face-centred).
By convention, the lattice vectors are named a, b and c and the angles are given the Greek letter corresponding to the lattice vector that is not spanning the angle, i.e. the angle between a and c is β.
The equivalent of the two-dimensional oblique lattice in three dimensions is the triclinic Bravais lattice. All angles are irregular and the three lattice vectors have different lengths. More symmetric lattices arise when some or all angles are 90° or 120° or when two or all three lattice vectors have the same length.
Among the lattices with exclusively right angles are the orthorhombic, tetragonal and cubic lattices depending on whether there are three, two or just one distinct lattice vectors in terms of their length. If only two angles are 90°, the cell is monoclinic, resulting in four rectangular and two skewed faces to the unit cell. If none of the angles is a right angle, the cell is trigonal if all lattice vectors are the same length but triclinic if they are different. If only one angle is skewed, the resulting cell is called monoclinic. Finally, the hexagonal lattice has one angle at 120° and two at 90°.
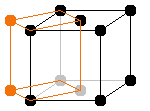
The gaps in the table arise because the missing lattices can be expressed in terms of one of the others by choosing a different unit cell. For example, a side-centred cubic lattice is the same as a primitive tetragonal one with a smaller unit cell based on four of the corner atoms and four side-centred atoms of the cubic cell.
Next (in the Concepts lecture), we'll discuss some common crystal structure types found in materials and minerals, and how they are structurally related to one another.
For a more in-depth view of crystal structures (in the Structure Determination lecture) we'll see how to deal with crystals containing more than one type of atom by introducing the wider concept of symmetry groups.