

Heat, $Q$, is a form of energy associated with temperature. Microscopically, it can be seen as the collective kinetic energy of all the atoms contained in a system. While everyday language doesn't distinguish clearly between heat and temperature, it is important to recognise the precise meaning of heat in thermodynamics as a form of energy (measured in J) related to temperature (measured in K).
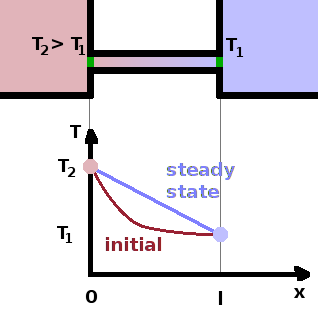
Exchanging heat with its surroundings is one way for a system in which to interact. To understand what happens during a heat transfer, we consider two reservoirs at different temperatures. A reservoir is a system which is very large, such that it contains enough energy to render insignificant any transfers of energy into or out of it. Therefore, a finite transfer of heat will not change the temperature of the reservoir. The two reservoirs under consideration are linked by a thermal conductor, so heat can flow from the hotter to the cooler reservoir - left to right in the figure.
Initially, as heat begins flowing from the hot reservoir along the conductor, the temperature measured along the conductor decreases sharply, but as, over time, heat spreads along the length of the conductor, a steady state develops, characterised by a uniform linear temperature gradient along the conductor. The time it takes to reach the steady state is a relaxation process in the same way that equilibration is. However, since the reservoirs are infinitely large, the temperature difference is maintained, preventing a thermodynamic equilibrium (i.e. uniform temperature). In equilibrium, state variables are equal; in a steady state they may be different but they do not change.
We can quantify the heat transfer from hot to cold in terms of the heat current $I$ (in W): $$I=\frac{Q}{\Delta t}$$ or, normalised to the cross section $A$ of the thermal conductor, as the heat flow $j$ (in W/m2) $$j=\frac{Q}{A\Delta t}\qquad.$$ The rate at which heat is transferred is proportional to the temperature gradient, so $$j=-\kappa\frac{T_2-T_1}{l}=-\kappa\frac{{\rm d}T}{{\rm d}x}$$ where the constant of proportionality is the thermal conductivity $\kappa$ (in W/(K m)).
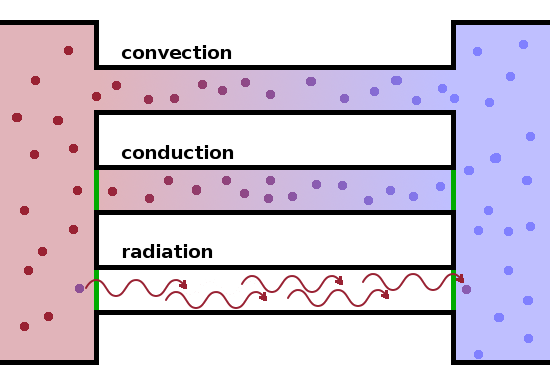
In addition to conduction, i.e. heat transfer through a medium but without associated mass transfer, heat can be transported through convection, where heat is transferred through the exchange of hot matter, and by radiation, where photons act as carriers of heat.
In the scenario above, we have allowed heat to be transferred from a reservoir to a thermal conductor attached to it. This means that the wall of the system at the point of contact has different characteristics from the rest of the wall, where we don't allow heat transfers.
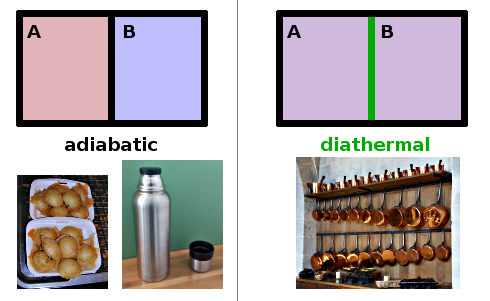
In thermodynamics, walls that allow heat to pass through while keeping matter apart are called diathermal walls. Two (finite) systems separated by a diathermal wall will equilibrate. A good approximation to a diathermal wall is a sheet of copper, given its high thermal conductivity. On the other hand, the vacuum of a thermos flask or the closed porosity of styrofoam prevent heat transfers. Such walls are known as adiabatic walls. Of course, no real material is perfectly diathermal or perfectly adiabatic - these two concepts are ideal types useful in modelling real behaviour in approximation. Both types of wall prevent exchange of matter; they enclose closed systems.

Given the distinction between these two types of wall, we can conceive of two systems (A,B in the figure on the left) separated by an adiabatic wall and therefore without thermal contact. If each of the two systems is in thermal contact (through a diathermal wall) with a third system (C), then each will equilibrate with that system. Since temperature, as a state variable, is uniform once equilibrium is established, the temperature throughout system C will be uniform, and therefore A and B will be in equilibrium via their interaction with C.
As this is fairly intuitive, it wasn't originally stated explicitly when the theory of thermodynamics was first developed and was later added as the "zeroth" law of thermodynamics. It is, however, conceptionally important because it hinges on the fact that temperature is a state variable and therefore independent of the process history of a system. A concise statement of the Zeroth Law is: Two systems in thermal equilibrium with a third system are in equilibrium with each other.
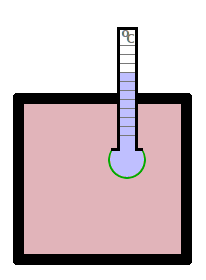
The thermometer is based on equilibration through diathermal walls: The tip of the thermometer must have good thermal contact with the system to be monitored, so the tip should ideally have a diathermal wall. On the other hand the shaft of the thermometer ought to be adiabatic as we don't want heat to be conducted away from the system studied. The thermometer really measures the temperature of the fluid contained in it rather than the temperature of the medium it is immersed in. It only works because equilibrium is established between the system and the thermometer fluid.

We have stated previously that it is necessary to distinguish between temperature (random kinetic energy contained in a system, in units of the Boltzmann constant, $k_B$) and heat (energy transferred due to differences in temperature). The reason for this becomes obvious when we consider the temperature changes during a phase change, such as boiling a kettle.
Below the boiling point, while all the water in the kettle is liquid, the system kettle is in a single-phase region. All the heat transferred into the system is used to heat up the liquid. The increase in temperature per unit of heat supplied is given by the heat capacity $c_p$ in J/(mol K), a material property characteristic of each substance and its given state of matter. It describes the (infinitesimal) amount of energy needed to (infinitesimally) increase the temperature of the substance: $${\rm d}Q_p=nc_p{\rm d}T$$ In practice, the temperature dependence of $c_p$ tends to be small so that it can often be treated as a material constant (as long as the substance doesn't change its state of matter), in which case the infinitesimal changes can be replaced by macroscopic differences. In engineering contexts, the specific heat in J/(kg K) is often used instead of the heat capacity, i.e. the quantity is normalised to the mass rather than the amount of material. The subscript $p$ in $c_p$ indicates that the heat is transferred at constant pressure. The heat capacity at constant volume, $c_V$, is generally smaller than $c_p$, particularly for gases.
As soon as the boiling point is reached, the system reaches a phase transition region. While there are two phases present (here, liquid water and vapour), all the heat supplied is used to drive the phase transition; the temperature remains constant while this happens. As there is no temperature change, the heat capacity of the material is irrelevant now. Instead, the latent heat $L$ in J/mol of the phase transition determines how much energy is needed to vapourise an amount of material: $$Q=nL$$ As with heat capacities, latent heats are sometimes normalised to a mass rather than an amount of material, particularly in engineering contexts.
If heat is the energy flowing due to the collective random movements of a medium, we need to distinguish from other forms of energy in transit which act in a particular direction. These other forms come under the heading of work.