[web] [lect]

This page contains a number of examples which resemble time-domain data obtained with a Fourier-transform spectrometer such as widely used for nuclear magnetic resonance (NMR) and infrared (IR) spectroscopy. These signals are typically exponential decays with several superimposed oscillations. The decay transforms into the line width, while the oscillations show up as line positions in the Fourier transform, i.e. the spectrum. If you have gnuplot or MathCAD or the like, you can try this at home; mp260's worksheet 5 gives instructions.

Single-exponential decay

A real-only single-exponential decay 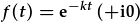f(t)= {\rm e}^{-kt}(+{\rm i}0)
transforms as F(\omega)=\frac{1}{2\pi}\int_{-\infty}^{+\infty}{\rm e}^{-kt}{\rm e}^{-{\rm i}\omega t}{\rm d}t.

If we let the time axis begin at zero and, from a point a onwards, the signal is practically indistinguishable from zero,

then the limits become 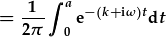=\frac{1}{2\pi}\int_0^a{\rm e}^{-(k+{\rm i}\omega)t}{\rm d}t.
Integrate: =\frac{1}{2\pi}\left[-\frac{1}{k+{\rm i}\omega}{\rm e}^{-(k+{\rm i}\omega)t}\right]_0^a,
substitute limits: 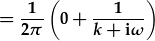=\frac{1}{2\pi}(0+\frac{1}{k+{\rm i}\omega})
(the first term is zero by definition of a as cut-off value).
Tidy up: 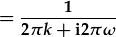=\frac{1}{2\pi k+{\rm i}2\pi\omega}.
To see what the real and imaginary parts of the solution are, it is better to get rid of the complex unit in the denominator. This can be done by multiplying both numerator and denominator by the conjugate of the denominator:
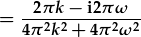=\frac{2\pi k-{\rm i}2\pi\omega}{4\pi^2k^2+4\pi^2\omega^2}.
Tidy up again: 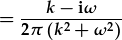=\frac{k-{\rm i}\omega}{2\pi(k^2+\omega^2)}.
Fig.: Single-exponential decay and its Fourier transform. Click image to Fourier transform. (Alternatively, view Fourier transform here.)
  • Compare two exponential decays, a slow one (k=1) and a fast one (k=2).
  • Note that the spectrum is complex although the time-domain data was real only!
  • The real part is a symmetric line while the imaginary part is antisymmetric. In spectroscopy, these shapes are known as absorption and dispersion lines.
  • Slower decay - narrower spectrum. This is an important property of Fourier transform pairs: When one increases, the other one decreases as they are reciprocal. Think Fourier!

Phase shifting

In the example above, the input function was real-only. In general, there will be a phase factor which determines how the intensity is distributed between the real and imaginary parts.

Decay function with phase factor: f(t)= {\rm e}^{-kt}{\rm e}^{{\rm i}\phi},
which is the same as 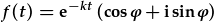f(t)= {\rm e}^{-kt}(\cos\phi+{\rm i}\sin\phi),
i.e. the real and imaginary parts are modulated by a cosine and sine wave, respectively.
The Fourier transform is: 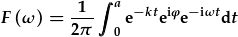F(\omega)=\frac{1}{2\pi}\int_0^a{\rm e}^{-kt}{\rm e}^{{\rm i}\phi}{\rm e}^{-{\rm i}\omega t}{\rm d}t.
The phase factor doesn't depend on t. So, 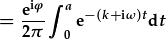=\frac{{\rm e}^{{\rm i}\phi}}{2\pi}\int_0^a{\rm e}^{-(k+{\rm i}\omega)t}{\rm d}t,
which contains the same integral as above: 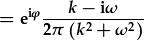= {\rm e}^{{\rm i}\phi}\frac{k-{\rm i}\omega}{2\pi(k^2+\omega^2)}.

This results in the following time-domain signals and spectra (click images to transform):

No phase shift.

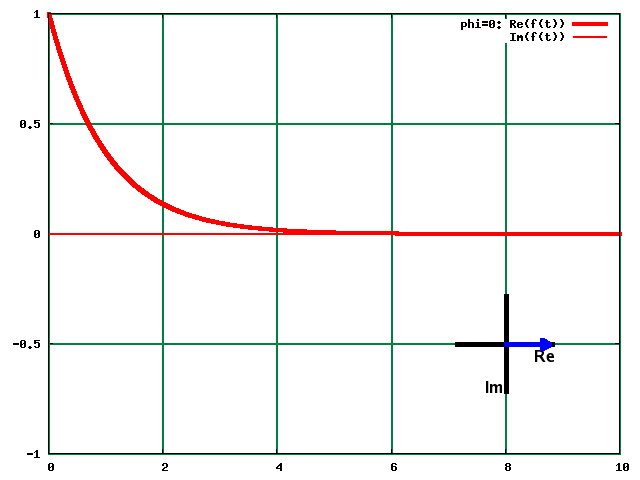Fig.: Purely real input function and its Fourier transform. Click image to Fourier transform. (Alternatively, view Fourier transform here.)
  • This is the original real-only input function again.
  • The insert shows the orientation of the data set in the complex plane.
  • Click on the image to Fourier transform.

phase=π/6

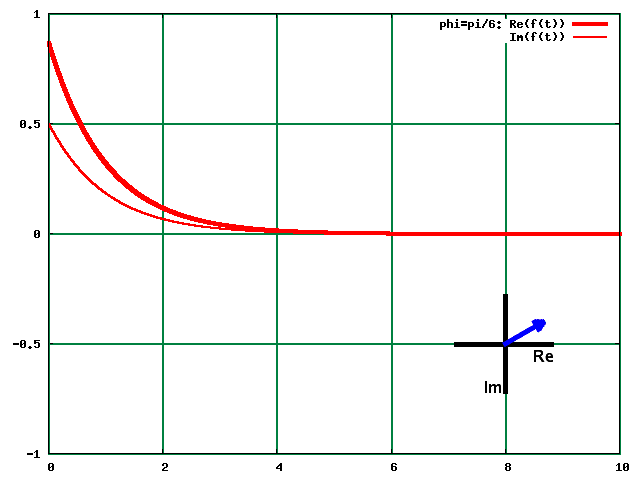Fig.: Function and its Fourier transform with a phase shift of pi/6. Click image to Fourier transform. (Alternatively, view Fourier transform here.)
  • As the phase angle increases, intensity is shuffled from the real part into the imaginary part and then back.
  • As a consequence, the line shapes aren't pure absorption or dispersion lines any more.

phase=π/4

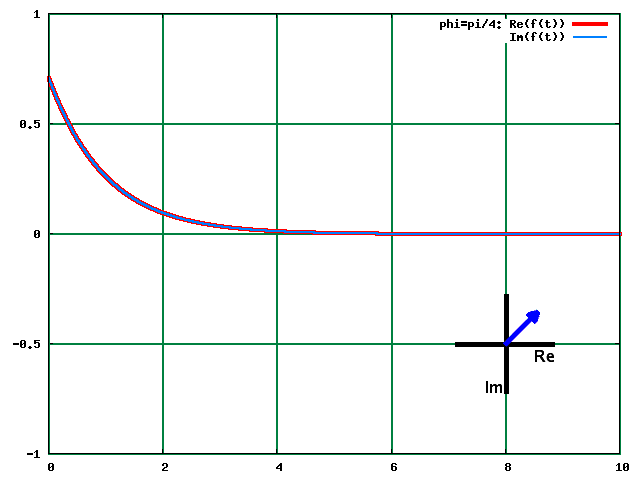Fig.: Function and its Fourier transform with a phase shift of pi/4. Click image to Fourier transform. (Alternatively, view Fourier transform here.)

At π/4, real and imaginary part of the input function are identical. In the corresponding spectrum, the real and imaginary lines are mirror images of each other. The mirror is the parallel of the y axis at the position of the line (here x=0).

phase=π/2

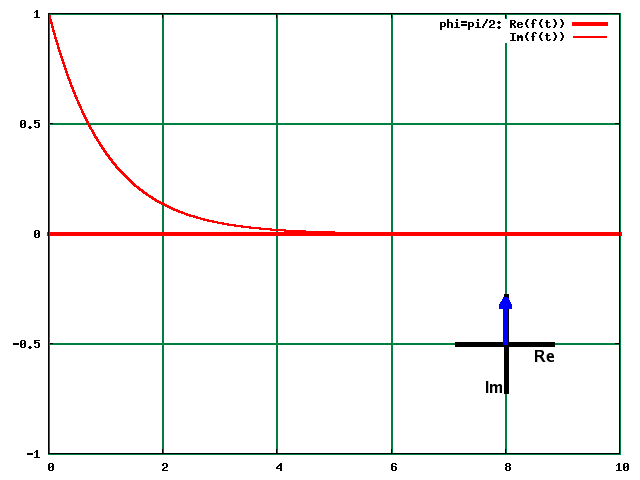Fig.: Function and its Fourier transform with a phase shift of pi/2. Click image to Fourier transform. (Alternatively, view Fourier transform here.)

At π/2, the real and imaginary parts are swapped w.r.t. the original function. In the spectrum, the two components swap also. Note that the real part now is a mirror image of the original imaginary part (because Im is always behind Re by π/2).

phase=π

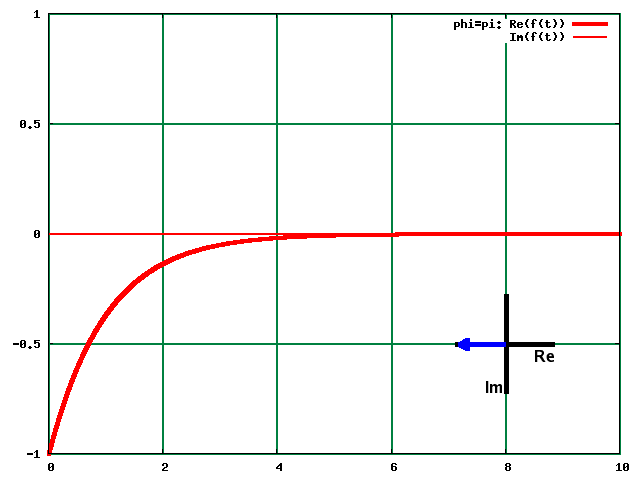Fig.: Function and its Fourier transform with a phase shift of pi. Click image to Fourier transform. (Alternatively, view Fourier transform here.)

At π, the real part is inverted; hence the spectrum is also inverted.

Slow and fast decays superimposed

The sum of two decays: 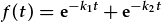f(t)= {\rm e}^{-k_1t}+{\rm e}^{-k_2t}
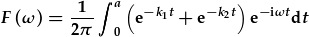transforms as F(\omega)=\frac{1}{2\pi}\int_0^a({\rm e}^{-k_1t}+{\rm e}^{-k_2t}){\rm e}^{-{\rm i}\omega t}{\rm d}t.
That integral can be split: =\frac{1}{2\pi}\int_0^a{\rm e}^{-(k_1+{\rm i}\omega)t}{\rm d}t+\frac{1}{2\pi}\int_0^a{\rm e}^{-(k_2-{\rm i}\omega)t}{\rm d}t
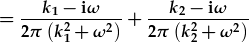into two of the usual form. Thus, =\frac{k_1-{\rm i}\omega}{2\pi(k_1^2+\omega^2)}+\frac{k_2-{\rm i}\omega}{2\pi(k_2^2+\omega^2)}.
Fig.: Double exponential decay and its Fourier transform. Click image to Fourier transform. (Alternatively, view Fourier transform here.)
  • This is an example of the addition theorem at work.
  • The fast decay contributes a broad spectral component; the slow decay causes a narrow component. Think Fourier!
  • In this example, the intensities (integrals) of both lines are the same, although it doesn't look like that. This is a common problem when interpreting spectra: broad lines tend to get overlooked, especially when masked by noise.

Damped oscillation

An oscillation is best described as a complex exponential. A damped oscillation is an exponential decay (as before) with an oscillation superimposed, i.e. multiplied to it.

So, the input function is: 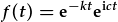f(t)= {\rm e}^{-kt}{\rm e}^{{\rm i}ct},
which transforms as: F(\omega)=\frac{1}{2\pi}\int_0^a{\rm e}^{-kt}{\rm e}^{{\rm i}ct}{\rm e}^{-{\rm i}\omega t}{\rm d}t.
Integrate: =\frac{1}{2\pi}[-\frac{{\rm e}^{-(k+{\rm i}(\omega-c))t}}{k+{\rm i}(\omega-c)}]_0^a,
substitute limits: 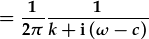=\frac{1}{2\pi}\frac{1}{k+{\rm i}(\omega-c)},
and tidy up 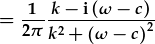=\frac{1}{2\pi}\frac{k-{\rm i}(\omega-c)}{k^2+(\omega-c)^2}.
Fig.: Damped oscillation and its Fourier transform. Click image to Fourier transform. (Alternatively, view Fourier transform here.)
  • The oscillation causes the spectrum to shift. The offset from the original line position is equal to the frequency of the oscillation.
  • While the decay determines the line width, the frequency determines the line position. This is why Fourier transformation is so useful in spectroscopy: From the line position we see at what frequencies (and hence energies) an interaction occurs, and from the line width we can draw conclusions about the range of the interaction. Finally, the intensity (integral) of the spectral line indicates the likelihood that the corresponding transition between energy levels occurs.
  • All this information is contained in the original time-domain data, but Fourier transformation helps retrieving it from the --usually messy-- raw data.

Two oscillations

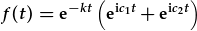In a system with two different energy transitions, photons of two different frequencies will be absorbed. Thus we have an exponential decay with two superimposed frequencies: f(t)= {\rm e}^{-kt}({\rm e}^{{\rm i}c_1t}+{\rm e}^{{\rm i}c_2t}).

According to the addition theorem, these give rise to two separate lines, each shifted by their frequencies c1 and c2, respectively.

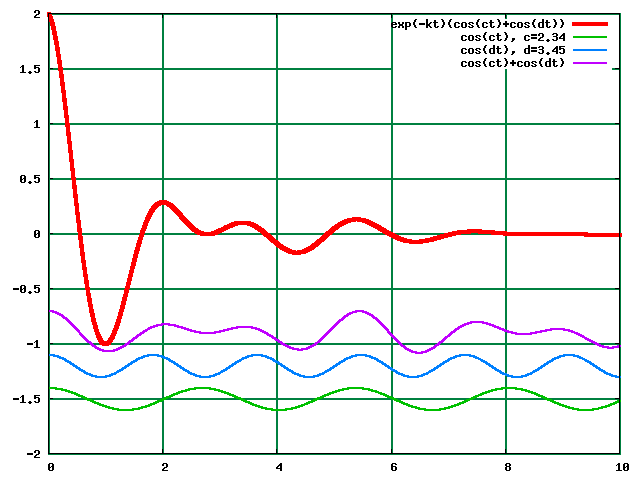Fig.: Damped double oscillation and its Fourier transform. Click image to Fourier transform. (Alternatively, view Fourier transform here.)
  • The input function contains two oscillations with frequencies c1 and c2; the two oscillations together form bunches. Even with just two oscillations and without noise, the original function looks quite messy.
  • The spectrum appears to consist of one broad line, although its peak is more rounded than usual. By plotting the two components separately, we see that the broad line is a superposition of two lines of the normal shape at the appropriate frequencies. Note that the maximum amplitude of the resulting spectrum is at neither of these frequencies but between them.
  • If the component frequencies aren't known, such line shapes need to be analysed by curve fitting.

Truncation: Fourier wiggles

Experimental data sets cannot continue forever. If a data set is truncated before all oscillations have petered out, the spectroscopist's pet artifact, the dreaded Fourier wiggles, are created.

So far, we have assumed that we can terminate the integration at some value a at which the signal becomes indiscernible from zero. Here's what happens if the input function drops to zero immediately, i.e. the data set ends prematurely.

Multiply the usual decay by a step function: f(t)= {\rm e}^{-kt}z(t),
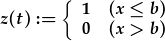where z(t):={\begin{array}1&(x\leq b)\\0&(x >b)\end{array},
where f(t) is still discernible from zero at t=b.
The Fourier transform is: 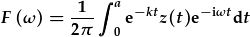F(\omega)=\frac{1}{2\pi}\int_0^a{\rm e}^{-kt}z(t){\rm e}^{-{\rm i}\omega t}{\rm d}t,
but z(t) forces f(t)=0 for t>b: 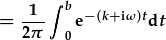=\frac{1}{2\pi}\int_0^b{\rm e}^{-(k+{\rm i}\omega)t}{\rm d}t.
Integrate: =\frac{1}{2\pi}[-\frac{{\rm e}^{-(k+{\rm i}\omega)t}}{k+{\rm i}\omega}]_0^b
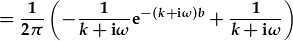
and substitute limits: =\frac{1}{2\pi}(-\frac{1}{k+{\rm i}\omega}{\rm e}^{-(k+{\rm i}\omega)b}+\frac{1}{k+{\rm i}\omega})
because this time, at t=b, f(t) hasn't decayed completely yet.
Tidy up: 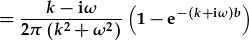=\frac{k-{\rm i}\omega}{2\pi(k^2+\omega^2)}(1-{\rm e}^{-(k+{\rm i}\omega)b}).
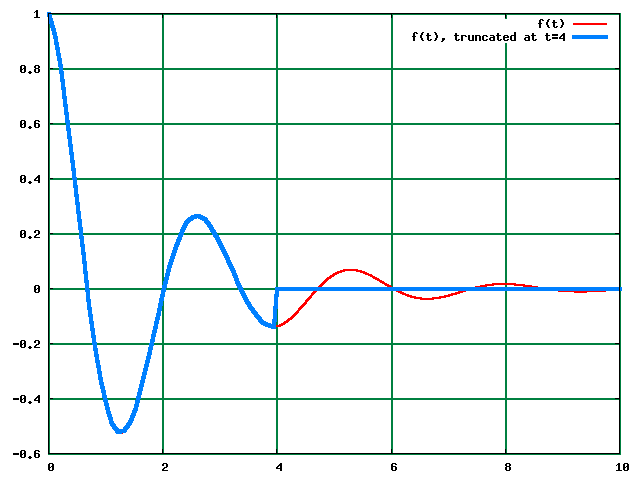Fig.: Truncated function and its Fourier transform. Click image to Fourier transform. (Alternatively, view Fourier transform here.)
  • The originating function is truncated at t=4 in this example.
  • The spectrum of the truncated function shows periodic wiggles on either side of the main line. Multiplying the unperturbed input function with a step function results after transformation in a convolution of the usual spectrum with the function sinx/x (also sometimes called sincx).
  • The Fourier wiggles do not affect the position of spectral lines, but they do affect the line width and intensity. If there are several lines present, Fourier wiggles belonging to a strong line can overlap with a weaker line, thereby producing an apparent shift of the latter.
  • In practice, the only way to avoid this artifact is to ensure data are recorded for sufficient time. If this isn't possible, the raw data can be multiplied by an exponential decay which forces the data down to virtually zero at the end of the data set. This suppresses the wiggles but can increase the line width.