Fundamental systems
The Schrödinger equation has been set up and solved for a number
of fundamental systems. The purpose of the fundamental systems is
to simplify solving the Schrödinger equation by focussing on only
one particular property of a real system at a time. In many cases, good approximate
solutions for real systems can be obtained by linear combination of a
small number of relevant fundamental systems. For example, in a water
molecule, we have two hydrogen atoms and another atom, which we can try to
treat as hydrogen-like to start with. In addition, there are two bonds
which can vibrate and the molecule can rotate around its two-fold symmetry
axis.
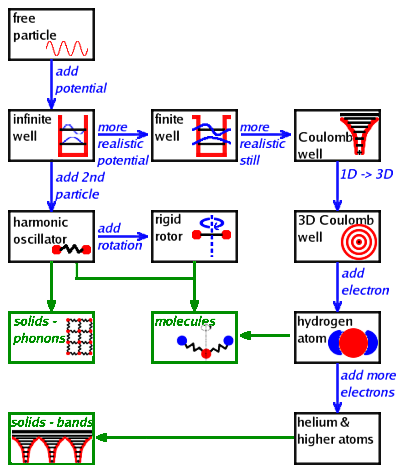
The fundamental systems:
-
Free particle. In the absence of any potential, the particle can move freely; its wave
function is a sine (or cosine or complex exponential) wave.
-
By confining the particle in an infinite well potential, a set of energy eigenvalues
(distinct states) emerges. Each state is characterised by a wave function whose period is such
that it is a standing wave within the width of the well.
-
If there is a finite potential drop, the particle's wave function is not forced to zero
at the boundaries of the well. Instead, the wave function decays exponentially outside the well,
which allows the particle to tunnel into a neighbouring well. This is exploited in the
scanning tunnelling microscope.
-
When dealing with atoms, it is more appropriate to use a Coulomb potential because of the
positive charge of the nucleus.
-
An atom is a three-dimensional Coulomb well, where the potential along the three Cartesian
coordinates is approximately the same, i.e. the atom is approximately spherical. Therefore,
it is worthwhile to switch to spherical coordinates at this point.
-
In a hydrogen-like atom, a nucleus and one electron share a Coulomb well. Instead of
dealing with the two masses independently, the reduced mass of the two objects and their common
centre of gravity is used when solving the Schrödinger equation of the
hydrogen atom.
-
More complex atoms are covered by including the inner electron shells together with the nucleus
in the atom core. These electrons do nothing but shield the positive charge of the nucleus.
-
When combining two atoms, we can take the wave functions calculated for each one of them and
add the properties of the chemical bond by taking into account the vibrational and rotational
motions of the molecule, e.g. in order to predict infrared and Raman spectra. The vibrations
are covered by the harmonic oscillator...
- ...and the rotations by the rigid rotor.
The harmonic oscillator can be developed further to explain the
vibrational states of solids (phonons),
while the combination of many atoms with their Coulomb potentials leads to the
electronic band structure
observed in solids. Molecules, on the other hand, are best described
by a combination of harmonic
oscillators (one for each bond) and rigid rotors (one for each symmetry axis).
Solving the Schrödinger equation
To solve the Schrödinger equation for a particular system, we need:
- a suitable potential
- a kinetic energy term
-
to decide which reference frame is best suited to the geometry of the problem (e.g. spherical
coordinates for spherical objects, axial coordinates for oblong objects such as chemical bonds)
- a trial wave function (a complex exponential is usually a good guess)
-
a set of boundary conditions (the Schrödinger equation is a
heterogeneous 2nd order partial differential equation with
3 independent variables; as such it needs up to 2x3=6 boundary conditions). These could be
nodes of the wave function at a potential step or a continuity condition when an angular variable
makes a full circle.
The solutions are pairs of wave functions and their energy eigenvalues.
Properties of a wave function
The wave function of a particle is a complex function (in the sense of having real and imaginary
parts). Its complex square (i.e. the product of the function and its complex conjugate)
is a real and positive function which represents the probability density of finding the
particle as a function of the spatial coordinates.
Therefore, only those solutions of the Schrödinger equation which satisfy the following conditions
are physically sensible:
- The wave function must be continuous.
-
It can be normalised. (The particle must be somewhere, so the integral of the
probability density over all space must equal 1.) That is to say, its complex square must be integrable.
- Its 1st and 2nd derivatives must also be continuous.
- It must have just a single value for each set of coordinates.

